The 4.79% odds of being dealt a blackjack is an average probability, based on no prior information. Of course if you were to keep track of the total number of cards dealt, including the exact number of ten-value cards & aces, you could calculate the precise odds before any given round. In the classic Blackjack, players need to make their decisions by only seeing one of the dealer’s cards which is called the up card. If by any chance, they get to see it, this places them in a very advantageous position and their chances of winning are significantly increased.
'What percentage of hands are suited blackjacks? Six-deck shoe, any suit.
RWR FROM TUSCON, USA
The probability of a suited blackjack in a six-deck game is 2*(4/13)*(6/311) = 0.0118723.'
So for 5 decks (not that it will change, but just do the math): 2*(4/13)*(5/259) = .0118, or 1.18%, all the same.
Be sure to leverage the search functionality, or even Google (all I did was type 'odds of getting suited blackjack' and the Wizards page was the first hit).
1-0.3051= 69.49%
This is for 100 repeated trials of taking cards out of a fresh shoe. I'm not sure what is meant by '5 deck shuffler', but if cards are discarded each hand, that will change your probability. That calculation would be rather cumbersome without programming.
About 1 in 4 of those will be suited.
This rough math gets you to 1/84=0.0119 chance of a suited blackjack, which is very close with much less math.
... which is very close with much less math.
Dieter, we're supposed to teach the kids that math is fun! =P
Dieter, we're supposed to teach the kids that math is fun! =P
It is! Math is great fun!
... but useful approximations are at least as fun, and quite useful, and often easier to remember.
Every schoolkid should know that (about) 1 hand in 21 should be a blackjack. If you play, that's just something you should know. It should be obvious that 1 in 4 will be suited.
As for accuracy... 1.187% vs 1.190% is darn close. 3 one thousandths of one percent close. Surely good enough for government work.
It's right up there with 'pi seconds is about a nanocentury'. (Of course, it's actually closer to 'square root of 10 seconds', but that's less fun.)
I'm not sure what is meant by '5 deck shuffler', but if cards are discarded each hand, that will change your probability. That calculation would be rather cumbersome without programming.
Based on how the question is phrased, I'd assume that the game is being played out of a 5-deck CSM. If you're not acquainted with them, they're pretty common machines in the US where the cards are fed back into the machine and reshuffled every few hands. Based on what I've seen, I'd estimate that, on average, ten cards will be in the discard at a given time. (Obviously, highly dependent on casino policy, number of hands, etc.)
It's close enough to playing with a constantly fresh n-deck game that the math shouldn't be thrown off by much though.
It is! Math is great fun!
... but useful approximations are at least as fun, and quite useful, and often easier to remember.
Every schoolkid should know that (about) 1 hand in 21 should be a blackjack. If you play, that's just something you should know. It should be obvious that 1 in 4 will be suited.
As for accuracy... 1.187% vs 1.190% is darn close. 3 one thousandths of one percent close. Surely good enough for government work.
It's right up there with 'pi seconds is about a nanocentury'. (Of course, it's actually closer to 'square root of 10 seconds', but that's less fun.)
Am I missing pi ~ 22/7 in that image? How could Randall forget that, or is it too obvious?
Am I missing pi ~ 22/7 in that image? How could Randall forget that, or is it too obvious?
355/113 is closer. 7 digits of accuracy in only 6 digits.
Or, 4*atan(1) (in radians).
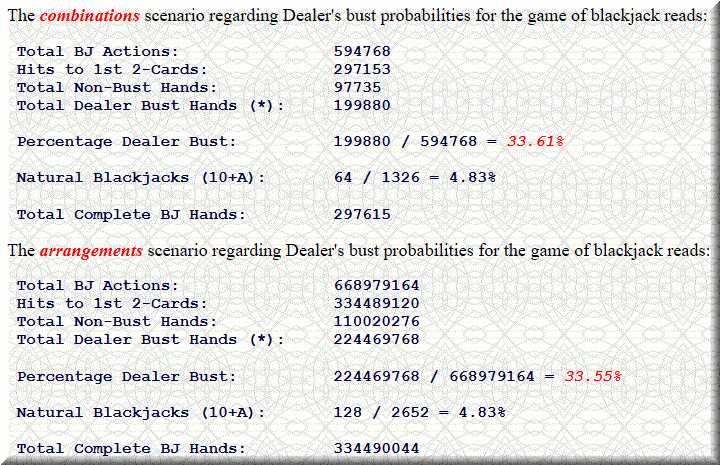
Blackjack Probability Worksheet
355/113 is closer. 7 digits of accuracy in only 6 digits.
Or, 4*atan(1) (in radians).
I agree 355/113 isbetter. 22/7 is just what we were told in some really basic math classes to use as an estimation.
Calculating Blackjack Probabilities
4atan(1) = pi, not an approximation
Probability Blackjack Questions
- Page 1 of 2